If you looking to hire expert that can help in your Java AI project then Realcode4you.com is the better choice. We are group of top rated Artificial intelligence experts and professionals that can help you to your advance Java Gamming projects.
Introduction
In this exercise, you will write a Java program to play the game of reversi, sometimes known by its trademark name of Othello. Reversi is played on an 8 by 8 board, initially set up as shown in figure 1, with the players moving alternately by placing their own pieces (black or white) on the board, one at a time. Black always goes first.

Figure 1: The opening position in a reversi game; black to move.
In the following description, a line segment is a sequence of board squares forming a contiguous straight line (horizontal, vertical or diagonal). The rule for a player to place a piece is:
The piece must be placed on an empty square such that there is a line segment passing through the piece played, then through one or more pieces of the opposing colour, and ending on a piece of the player’s own colour.
When such a line segment exists, we say that the opponent’s pieces on that line segment are bracketed. When a piece is played, bracketed pieces change colour according to the following rule:
For every a line segment passing through the piece played, then through one or more pieces of the opposing colour, and ending on a piece of the player’s own colour, the pieces of opposing colour through which that line segment passes are all changed to pieces of the player’s own colour.
In figure 2, the left-hand picture shows a possible move for white, which brackets three of his opponent’s pieces, resulting in the position shown in the right-hand picture.
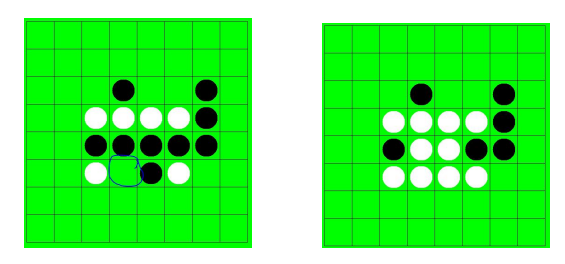
Figure 2: A move by white in a reversi game, and its result.
If, and only if, a player cannot move, but his opponent can, he misses a turn. The game ends when neither player can move. (This usually, but not always, happens because all the squares have been occupied.) The winner is the player with the greater number of pieces of his own colour on the board; if there is no such player, the result is a draw.
The main game logic is in the class BoardState. An instance of this class represents a situation in the game. The field colour encodes whose turn it is (1 for white; -1 for black), while the low-level methods int getContents(int x, int y) and void setContents(int x, int y, int piece) allow retrieval and setting on the invididual board squares. Here, a value of 1, -1 or 0 represents presence of, respectively, a white piece, a black piece or no piece at all at the square on rank x and file y. To make things easier for you, we have included the method boolean checkLegalMove(int x, int y), which checks whether it is possible for the current player to move on square (x,y), and void makeLegalMove(int x, int y), which actually executes the move. In fact, to make things really easy, we have provided a method to return the list of all and only those legal moves for the current player. Here, we rely on a class Move to encode the actual moves; it has just two public fields, x and y; so that instances of this class represent moves (legal or illegal) in the obvious way. The method for retrieving the list of legal moves for the current player is ArrayList getLegalMoves(). (Make sure you understand generic types in Java!). Notice that this list may be empty, because the current player may be unable to make a move.
The computer player is located in the class MoveChooser.java, of which the main program creates an instance. The only thing that this class does is implement the static method Move chooseMove(BoardState boardState). This is the method called when it’s the computer’s turn to move in the board state boardState. In its current version, this method just gets the legal moves and returns either null if that list is empty (remember what I said about there sometimes being no legal moves), and the first move in that list otherwise. The rest of the control is handled by the program. All you have to do is write a better move selection function than just picking the first. In fact, you must use minimax with αβ-pruning. The depth of the search should simply be controlled by the static final int searchDepth, which is currently set to 6 at the start of the Othello class. You may wish to set it to a lower value to speed up development. When you get everything working, try setting the search depth 8. This should be enough to thrash most human players, and won’t be too slow.
You will require a static evaluation function and there several choices available. For this assignment you need to desing yours as follows. Each square in the playing area is assigned a number:
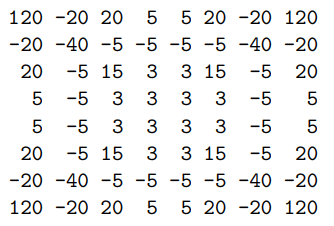
These numbers reflect the value for a player of being on the respective square. Note that the squares on the edges have high value (since pieces here are hard to take) and squares in the corners have an even higher value (since pieces here cannot be taken). By contrast, neighbouring squares have negative values, since a piece here will allow one’s opponent to move onto a high- value square. The value of a board position can then be defined by adding up the weights of all those squares occupied by white pieces and subtracting the weights of those squares occupied by black pieces. Thus, the value is always counted from white’s point of view. (Incidentally, the above array of values was taken from an older textbook by Peter Norvig).
You will also have to write a program to calculate minimax values of board positions. If you find αβ-pruning hard, you may prefer first to try ordinary minimax, and then graduate to αβ-pruning once that’s working. It is worth thinking a bit about what you will need to do when searching through a tree of board positions. Remember that instances of BoardState are Java objects. When you call BoardState.makeLegalMove(x, y), that will update the board (and the current player). If you want to get the daughters of a vertex boardState in the search tree, therefore, you will need to create, for each legal move, a fresh copy of boardState, and then execute the move in question on that copy. The method Boardstate.deepCopy(), which we have provided in BoardState is a cloning method, so that a call boardstate1 = boardstate.deepCopy() sets boardState1 to be a fresh copy of boardstate; subsequently modifying boardstate1 (e.g. by executing a legal move) does not then change boardstate. When computing the daughters of a vertex in the game tree, be careful about two corner cases: one is where the computer has no legal move. In that case, control passes to the other player. In terms of the search tree, this means that the vertex representing the board state in question has a single daughter, with all the pieces the same, but the current player changed.
The top-level call is a little different to subsequent calls, because you have to select the move that yields the best daughter (from the point of view of the computer player), rather than simply evaluating the daughter. It is important that, when and only when there are no moves available, you return the move null. This is fine: our code will understand what that means.
Try the program Othello.java out with your version of MoveChooser.java, and with the constant Othello.searchDepth set to the value 6. The resulting program should play a decent game of Othello against a human, and should certainly beat a player choosing moves more or less at random. Moreover, the program should not take more than about a few seconds for any move when run on an ordinary PC at that search depth; if so, you have probably written inefficient code.
To get solution of above problem you can contact us:
realcode4you@gmail.com
We are providing all advance gamming projects or assignments related help. To get help in other Java AI related help you can send your project requirement details here so we can provide quality code without any plagiarism issues.
Comments